We know that (xy) 3 can be written as (xy)(xy)(xy) We know that (xy)(xy) can be multiplied and written as x 2xy yx y 2 (xy) = x 22xy y 2 (xy) = x 32x 2 y xy 2yx 2 2xy 2y 3 = x
(x+y)^3 identity class 9-Formula ( x a) ( x b) = x 2 ( a b) x a b x is a variable, and a and b are constants The literal x formed a binomial x a with the constant a, and also formed another binomial x b with18/9/21 (i) To prove x 3 y 3 = (x y) (x 2 – xy y 2) Now, using identity VI we can say (x y) 3 = x 3 y 3 3xy (xy) Or, (xy) 3 – 3xy (x y) = x 3 y 3 Or, x 3 y 3 = (x y) {(x y) 2 – 3xy}
(x+y)^3 identity class 9のギャラリー
各画像をクリックすると、ダウンロードまたは拡大表示できます
 |  |  |
 |  | |
 |  |  |
 |  | |
「(x+y)^3 identity class 9」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  | |
 |  |  |
 |  | |
「(x+y)^3 identity class 9」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  |  |
 |  | |
 |  |  |
「(x+y)^3 identity class 9」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  | |
 |  |  |
 |  |  |
「(x+y)^3 identity class 9」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  |  |
 |  | |
 |  |  |
「(x+y)^3 identity class 9」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  |  |
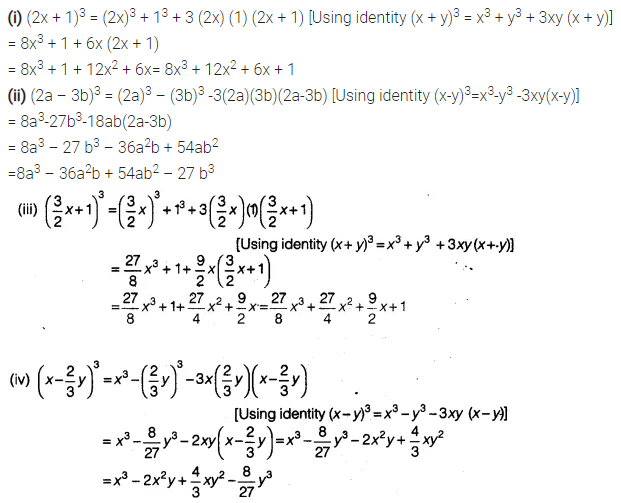 |  |  |
 |  |  |
「(x+y)^3 identity class 9」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  |  |
 |  | |
 |  | |
「(x+y)^3 identity class 9」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 |  | |
 |  |  |
 |  |  |
「(x+y)^3 identity class 9」の画像ギャラリー、詳細は各画像をクリックしてください。
 |  |  |
 | 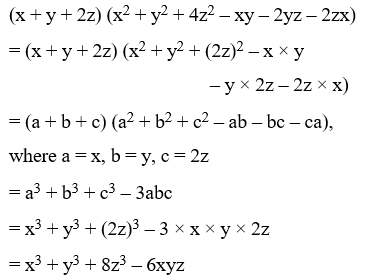 |  |
 |  |
9/7/21 Hope the information shed above regarding NCERT MCQ Questions for Class 8 Maths Chapter 9 Algebraic Expressions and Identities with Answers Pdf free download has been usefulSolution By the algebraic identity, x 2 – y 2 = (x y) (x – y), we can write the given expression as;
Incoming Term: (x+y)^3 identity class 9,



